replicating omega frequency | Angular frequency replicating omega frequency Related Reading:• Olenick, Richard P.; Apostol, Tom M.; Goodstein, David L. (2007). The Mechanical Universe. New York City: Cambridge University Press. pp. 383–385, 391–395. ISBN 978-0-521-71592-8. See more To search your Amazon order history, open Amazon on the web and click "Returns & Orders" in the top right corner. Just above your list of previous orders, you'll see a search box. Enter your search terms in this box and click "Search Orders," or hit Enter.
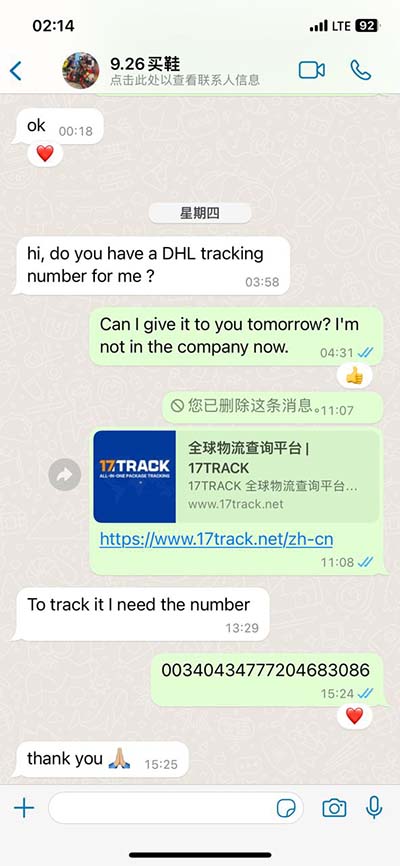
0 · trigonometry
1 · sampling
2 · Why are angular frequencies $\\omega=2\\pi f$ used over normal
3 · What is the significance of angular frequency $\omega$ with
4 · Mechanisms of Theta Plasmid Replication
5 · Is it real? A buyer's guide to spotting a fake Omega
6 · Angular frequency
7 · Amplitude, Frequency, Wave Number, Phase Shift
8 · 9.2: DNA Replication
9 · 6.02 Practice Problems: Modulation & Demodulation
Puerto Rico's Favorite Drinks packages Malta India Light and Malta India Regular 8 Fl Oz (12 Pack) Malta Mix 8floz. Options: 2 sizes, 10 flavors. $2471 ($2.06/Fl Oz) Typical: $26.41. Save more with Subscribe & Save. FREE delivery Wed, Jun 5 .
In physics, angular frequency (symbol ω), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine function (for example, in oscillations and waves). Angular frequency (or . See more
In SI units, angular frequency is normally presented in the unit radian per second. The unit hertz (Hz) is dimensionally equivalent, but by convention it is only used for frequency f, . See more
Although angular frequency is often loosely referred to as frequency, it differs from frequency by a factor of 2π, which potentially leads confusion when the distinction is not made clear. See moreRelated Reading:• Olenick, Richard P.; Apostol, Tom M.; Goodstein, David L. (2007). The Mechanical Universe. New York City: Cambridge University Press. pp. 383–385, 391–395. ISBN 978-0-521-71592-8. See moreCircular motionIn a rotating or orbiting object, there is a relation between distance from the axis, $${\displaystyle r}$$, tangential speed, $${\displaystyle v}$$, and the angular frequency of the rotation. During one period, See more• Cycle per second• Radian per second• Degree (angle)• Mean motion• Rotational frequency See more
trigonometry
To get the frequency domain representation of this modulated signal, we replicate the spectrum of the original signal at \(-\Omega_c\) and \(+\Omega_c\), scaling each of these replications by .
I wanted to "rediscover" the replication of the signal spectrum by pure algebraic manipulation from ejθ or cos(θ + ϕ). First you have to understand where images come from. . It's just nicer to name \pi f$ term as angular frequency $\omega$,- as it gives dimensions $[\text{rad/s}] \equiv [\text{rad} \cdot \text{Hz}]$. That's why it is sometimes called .
The angular frequency is related to a quantity often labeled f f and also called the frequency by \omega = 2\pi f ω = 2πf. With these new definitions, solutions to the wave equations can be . The frequency of replication initiation is tightly regulated to facilitate establishment in permissive hosts and to achieve a steady state. The last section of the article reviews how .
sampling
You could consider omega to be a pure indicator of periodicity in the cycle. Larger omega gives you more rads per second. Larger omega gives you shorter wavelength, and .
The reason we use the angular frequency, $\omega$, is because the \pi$ is always present and so to know how quickly the function repeats, i.e. to have an intuitive idea . The replication of DNA occurs during the synthesis phase, or S phase, of the cell cycle, before the cell enters mitosis or meiosis. The elucidation of the structure of the double .It should have a unique identifying serial number engraved on the case, which you can check against Omega's records. Fake Omega watches are among the most common kinds of .In physics, angular frequency (symbol ω), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase .
Why are angular frequencies $\omega=2\pi f$ used over normal
To get the frequency domain representation of this modulated signal, we replicate the spectrum of the original signal at \(-\Omega_c\) and \(+\Omega_c\), scaling each of these replications by . I wanted to "rediscover" the replication of the signal spectrum by pure algebraic manipulation from ejθ or cos(θ + ϕ). First you have to understand where images come from. .
Both processes are given from the shift property of the Fourier Transform which states that multiplying a function $x(t)$ in the time domain by $e^{j\omega_c t}$ will shift the . It's just nicer to name \pi f$ term as angular frequency $\omega$,- as it gives dimensions $[\text{rad/s}] \equiv [\text{rad} \cdot \text{Hz}]$. That's why it is sometimes called .
The angular frequency is related to a quantity often labeled f f and also called the frequency by \omega = 2\pi f ω = 2πf. With these new definitions, solutions to the wave equations can be .
The frequency of replication initiation is tightly regulated to facilitate establishment in permissive hosts and to achieve a steady state. The last section of the article reviews how .$\omega$: Normalized radian frequency. $\omega = \Omega/F_s = 2\pi F/F_s$. Sometimes its units are listed as being radians/sample. Because of aliasing, it is only necessary to study the . You could consider omega to be a pure indicator of periodicity in the cycle. Larger omega gives you more rads per second. Larger omega gives you shorter wavelength, and . The reason we use the angular frequency, $\omega$, is because the \pi$ is always present and so to know how quickly the function repeats, i.e. to have an intuitive idea .
In physics, angular frequency (symbol ω), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase .To get the frequency domain representation of this modulated signal, we replicate the spectrum of the original signal at \(-\Omega_c\) and \(+\Omega_c\), scaling each of these replications by . I wanted to "rediscover" the replication of the signal spectrum by pure algebraic manipulation from ejθ or cos(θ + ϕ). First you have to understand where images come from. . Both processes are given from the shift property of the Fourier Transform which states that multiplying a function $x(t)$ in the time domain by $e^{j\omega_c t}$ will shift the .
What is the significance of angular frequency $\omega$ with
It's just nicer to name \pi f$ term as angular frequency $\omega$,- as it gives dimensions $[\text{rad/s}] \equiv [\text{rad} \cdot \text{Hz}]$. That's why it is sometimes called .The angular frequency is related to a quantity often labeled f f and also called the frequency by \omega = 2\pi f ω = 2πf. With these new definitions, solutions to the wave equations can be .
The frequency of replication initiation is tightly regulated to facilitate establishment in permissive hosts and to achieve a steady state. The last section of the article reviews how .
$\omega$: Normalized radian frequency. $\omega = \Omega/F_s = 2\pi F/F_s$. Sometimes its units are listed as being radians/sample. Because of aliasing, it is only necessary to study the . You could consider omega to be a pure indicator of periodicity in the cycle. Larger omega gives you more rads per second. Larger omega gives you shorter wavelength, and .
Mechanisms of Theta Plasmid Replication
Is it real? A buyer's guide to spotting a fake Omega
Activer/désactiver l'historique de navigation. Si vous désactivez votre historique de navigation, les nouveaux articles que vous consultez ne s'afficheront pas sur cette page .
replicating omega frequency|Angular frequency